Theory Without Tears

The very word “theory” conjures up images of geometry class or, worse, physics lab. Scary stuff. Scarier, when you put the word “music” in front. “Music theory?” Music is supposed to be fun, carefree, an audio expression of our feelings. Music is not science. Is it?
Well, not rocket science, anyway (though the ancient Greeks did study harmony as part of the school of mathematics). We can nit-pick ourselves to death with this question – after all music is sound and sound is physics (again? arrrrgggg!). But let’s save ourselves trouble and anxiety by approaching music theory, and especially how it relates to the guitarist, with a simple idea: music theory is actually simple and fun. Okay, it’s not really simple, but it’s nowhere near as complicated as you might think. And it really is a lot of fun. In the introduction of Harmony by Walter Piston (possibly the best music theory text a person could ever hope to have), we read:
“…musical theory is not a set of directions for composing music. It is rather the collected and systemized deductions gathered by observing the practice of composers over a long time, and it attempts to set forth what is or has been their common practice. It tells not how music will be written in the future, but how music has been written in the past.”
Over the course of future columns, we will cover quite a bit of theory, sometimes when you least expect it. In this column, though, I’d like to go over the formation of chords.
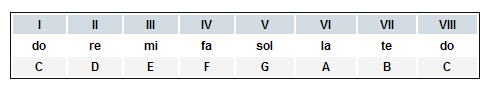
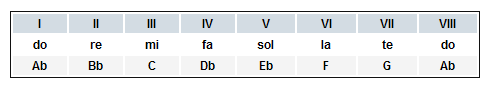
First, we need to get on same page, so to speak. Virtually everybody seems to call musical terms by different names, so let’s quickly agree on a few things. The major scale, for instance. Whether you still use the “Sound of Music” theory (“Doe, a deer…”) or various numbering schemes, I think we will all concede that the major scale is eight notes (although we will argue later about whether it’s seven or eight…).
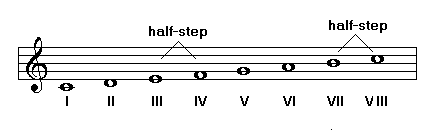
Starting with the first note, we take a whole step to reach the second and then another whole step to get to the third. This is followed by a half step, then three more whole steps and then a final half step (remember that each fret on the guitar is a half step). Using the key of C (as pictured), the scale is as follows:
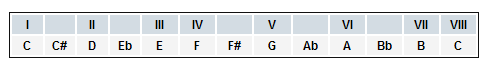
We know that there are other half steps lurking in there as well. These are our “sharps” (indicated with a “#” sign) and “flats” (indicated with a “b” sign). You’ll learn later that these notes share names. C#, for instance is the same as Db. Most notes are a whole step apart, with the exception of E and F and B and C, which are only a half step apart from each other. So, if we were to write these half steps into our chart, the notes (with the steps of the major scale still in place) would look like this:
(And while we’re agreeing on things, let’s also agree on this: the “I” note is called the “root” or “tonic.” This is to ensure that we are all starting with the same “do” when constructing our scales. Using this same chart and “rotating” either the notes or the Roman numerals in one direction or another, I can figure out any major scale in any key.
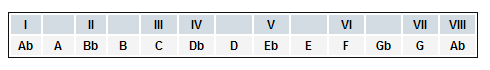
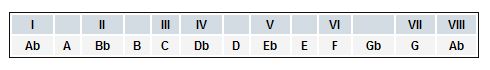
Pick a key, any key. How about A flat?
In realigning the notes, Ab is now the root (“I”) of the scale. So that is where we start. First we put out all the notes (in half steps) and then place the Roman numerals in their proper place (whole step, whole step, half step, whole step, whole step, whole step, half step). This is what you should have:
And finally, I remove the notes that do not have Roman numerals above them and voila! – a scale in A flat major:
Okay? Let’s move onto forming chords.
Now, and indulge me in this, imagine that you are the leader of a small choir. “How small is it?” There are only six voices in your choir. Okay? This is your guitar. Each string is one voice and each voice can only “sing” one note at a time. You chose whether one note is being sung at a time or whether (and which notes) all six voices are sounding at once. If you strike two or more separate strings, but they sound the same note (playing the fifth fret of the A string and an open D string, say), then the voices are in unison. If they are sounding different notes, you are playing a chord. Maybe. A chord must be at least three different notes – two different notes are not a chord. Let’s see:
The first group of notes are E (second fret on the D string) and G (open G string). It is impossible to call them a chord. They could be a part of many chords – think about how many simple chords you know that use these notes. E minor works. So does A7, A minor 7, A 11 (that’s the second chord in the song “Spooky,” in case you want a reference), C, C major 7, F major 7 add9, D sus 4 add 9, and on and on. It is not until we add the C note (at the bottom of the second group) that we have a definite chord. Now, this could also be part of a larger chord, such as A minor 7 or F major 7 add 9, but it is recognizable as a chord on its own.
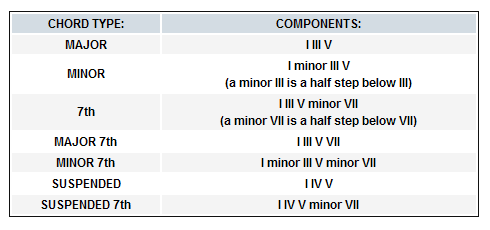
Believe it or not, you are now equipped to figure out chords all by yourself. All you need to know is how any particular type of chord is constructed. Let’s go over the most basic ones.
Okay, can we make an Ab minor chord? Well, let’s consult our Ab scale again (for simplicity’s sake I’m going to use the full scale with all the half steps):
So you can see that an Ab minor chord would consist of Ab (I), B (minor III) and Eb (V).
Now, even if you’d never played a particular chord before, you should be able to figure it out pretty handily. Let’s try a D minor 7th, okay? First, we construct our D scale. From this we’ll see that for this chord we’ll need D (I), F (minor III), A (V) and C (minor VII). D is sounded with, of all things, the open D string. Covering the second fret of the G string will give us the A note and if we place a finger on the first fret of the high E and B strings, then we’ll have the F and C as well. Ta da!
One last bit of advice: write things out! It really helps your thought process when you set stuff out on paper, one step at a time. As you get more proficient with practice, you’ll find it gets easier every time. Using this system will also help you when you start transposing and wait ’til you see how your knowledge of chord formation removes a lot of the mystique of alternate tunings. We’ll also be delving into “chord theory” to see how most song writing falls into fairly easily recognized patterns. We’ve got a lot of fun ahead of us!
Finally, let me add that this column is meant to be a very general overview, something to (a) get you started on music theory and (b) show you that music theory is truly painless. If you can count to twelve and keep your head, you’ll be in good shape. If you want to start delving into more, then look at some of the other articles here at Guitar Noise, such as the “basic theory trilogy” of The Musical Genome Project , The Power of Three and Building Additions (and Suspensions), or any of the terrific articles you can find on our music theory page.
Please feel free to bookmark this lesson for easy reference for your own lessons. If you have any questions, just send me a line or drop in on the Guitar Forums and get great feedback from your fellow guitarists. Guitar Noise offers numerous links to quite a few web sites that deal with music theory at all levels. Take the time to explore these and all the other tips, lessons and discussions that are here for your education and enjoyment.


Kristen
August 10th, 2015 @ 4:21 pm
This is GREAT! Thanks for posting!
Ari
April 8th, 2012 @ 6:08 pm
Hi, I hope you see this comment.
I was wondering, if with the chart you have, how one would annotate for chords that are between each roman numeral.
There’s a song that changes from Gm to G# to Cm, and I am unsure how one would represent that with roman numerals.
Scart
November 10th, 2016 @ 1:04 pm
see this link – it makes more sense.
http://mugglinworks.com/chordmaps/mapBb.htm
I would analyze it in the relative major key of Bb.
the progression is – vi – bvii – ii (that’s 6 flat7 and 2)
The middle chord is likely Ab instead of G# (and possibly just a bass note without implied harmony)
Hope this helps