Counterpoint – Part 2
Now that we’ve got the basic terminology behind us, on to writing counterpoint melodies.
The big breakthrough for Fux was dividing counterpoint lines into what he called “species”. In Fux’ view, there are five species of counterpoint:
- Note against note
- Two notes against one note
- Four notes against one note
- Offset melodies creating suspensions
- “Florid” counterpoint, consisting of all the others
Besides dividing an individual counterpoint into species, Fux divided counterpoints by the number of voices presented. In other words, he teaches first through fifth species counterpoint in two voices, then first through fifth species in three voices, and then he uses a fourth voice for all the species.
The species approach to counterpoint is used by most (but not all) counterpoint teachers. The alternative approach is called “direct” counterpoint. The logic of direct counterpoint says “Hey, it’s going to take me years to master each additional voice – how can I write for eight or ten voices right now, like Palestrina did?”.
But I’m not going to go there. For one thing, the guitar doesn’t have eight or ten strings. For another, I’ve read all these very academic texts, but I’ve never actually written eight or ten part counterpoint. So I’ll just show you what I actually know.
Counterpoint is traditionally done with a very slow melody – typically whole notes. There are a couple of reasons for that. First, it makes it easy to actually hear what third species (four notes against one, or four quarter notes against a whole note) sounds like; if you used sixteenth notes against a quarter note, it might become a sonic blur. The second reason is traditional: counterpoint originated as an alternative melody set against a Gregorian chant.
I’m not going to do that to you, because you probably aren’t writing Gregorian chant.
But I am going to start with first species two-voice counterpoint, because it gives us a simple foundation we can build on.
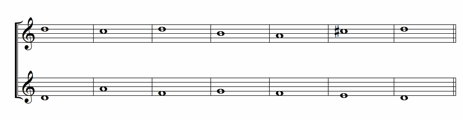
We’ll start with a simple melody. I’ll use D-A-F-G-F-E-D for our starting melody:
This starting melody is called the “cantus firmus” (literally, the “fixed song”), abbreviated CF. Against it, we’ll create the “contra punctus”, our counterpoint – abbreviated CP.
Our first decision is whether the CP should be above or below the CF. Whichever you choose, it should stay there – if your CP is below the CF, it should never rise above the fixed melody at any point; doing so is called “voice crossing”. Although voice crossing has been used in many pieces (even by famous counterpoint composers like Bach), it’s not a beginner technique, so we’ll set that as a hard rule for now.
The next question to answer is what note the counterpoint should start on. We’ve got a beginner rule for this too: if the CF is going to be above the CP, the CP is the same pitch – it’s either exactly the same pitch (a unison interval), or it’s one octave below. If the CP is set above the CF, you have two choices: the same pitch (at the unison or the octave), or a perfect fifth above the first note of the CF.
These choices ensure a solid beginning. The ending is also fixed: the last note should be the same letter name as the CF, either in unison or at the octave.
Next we need to pay attention to the final “cadence”, the point of tension that leads into the last note. In strict counterpoint, you don’t even need to think about this one: if the CF is the lower voice, the second-to-last harmony will be a major sixth above it; if the CF is the upper voice, the penultimate (I love that word!) harmony will be a minor third below it.
It’s the in between stuff we need to worry about. Fux created a set of four rules for counterpoint. Beethoven reduced them to two rules. I’ll do old Ludwig one better, and set just one rule for counterpoint: never move into a perfect consonance by similar motion.
Let’s apply this simple rule to our CF. In this example, I’ll put the CP above the melody, and start with an octave interval. I’ll also end with an octave, and since the second-to-last interval is fixed by the rules we have so far, our harmony looks like this:
Notice that our second-to-last harmony note is C#. There is no requirement that your counterpoint stay in the same key!
Now let’s dig a little deeper and see what I’ve done with the counterpoint. Here’s the last example with the intervals labeled (P=perfect, M=major, m=minor):
All of the intervals I used were thirds, sixths, or octaves. Fifths would also be allowed, but in first species every interval should be consonant – either perfect consonances or imperfect ones.
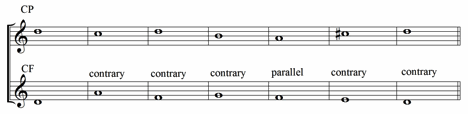
And here’s the same example one more time, with the motions labeled:
Notice that I’ve used mostly contrary motion. That allows both lines to sound independent. I also didn’t use any oblique motion… that would have been allowed. I could also have started with a perfect fifth interval. The various combinations would all sound pretty good; you’re limited a bit by the application of the rules, but the rules result in things that sound pretty good, so they allow us to build from a solid foundation. I’ll start showing you how to break the rules a few installments down the road.
Tom (“Noteboat”) Serb is a longtime Guitar Noise contributor and founder of the Midwest Music Academy in Plainfield, Illinois. This advice first appeared in Volume 4 # 26 of Guitar Noise News. Sign-up for our newsletter to receive more free tips like this by email.